| ECONOMICS OF TRUSSES AND GIRDERS | 177 |
|
pressure. A greater depth, if permissible, would have caused a saving in total weight of metal. In another of his designs for a five-hundred-and sixty-foot span a truss depth of ninety feet was adopted, but in this case the live load was very great, varying from ten thousand pounds per lineal foot for short spans to eight thousand pounds per lineal foot for long ones; and the bridge is twenty per cent wider than in the case of the two five- hundred-foot spans just mentioned. The greater the live load and the wider the bridge, the greater generally can the truss depth be made advantageously. The little mathematical investigation given in this chapter can be applied with fair accuracy to plate-girder bridges and to the floor systems of truss-bridges. If, for ordinary cases, in designing plate girders, one will adopt such a depth as will make the total weight of the web with its splice- plates and stiffening angles about equal to the weight of the flanges, he will obtain an economically designed girder, and a deep and stiff one. For long spans, however, this arrangement would make the girders so deep as to become clumsy and expensive to handle; consequently, when a span exceeds about forty feet, the amount of metal in the flanges should be a little greater than that in the web; and the more the span exceeds forty feet the greater should be the relative amount of metal in the flanges. The true economic investigation for plate-girders is as follows, when the web is assumed to resist its share of the bending moment: Let M = bending moment at mid-span, h = depth of web, t = thickness of web, S = intensity of working stress for tension, l = length of span, and c = ratio of weight of details of web (i.e., end stiffeners, intermediate stiffeners, splice plates, and fillers) to weight of the web plate itself. The sum of the two flange areas at mid-span, including an allowance of fifteen per cent for rivet holes, will be given by the equation, |
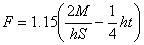 | [Eq. 4] |
|
and the total weight of metal in the flanges, taking into account the fact that the cover plates do not run the full length of the girder, will be given approximately by the equation, |
 | |
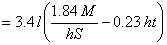 | [Eq. 5] |
|
The weight of the web and its details will be |
 | [Eq. 6] |